Adding and subtracting with exponents

In this case the two exponents 3x 2 and 7y 2 are unlike terms and so it will remain as it is. 4x12 3x12 025cdot x4 2x2 3x2 x12 025cdot x4 x2.

Order of Operations Factors Primes Fractions Long Arithmetic Decimals Exponents Radicals Ratios Proportions Percent Modulo Mean Median Mode Scientific Notation Arithmetics Algebra Equations Inequalities System of Equations System of Inequalities Basic Operations Algebraic Properties Partial Fractions Polynomials Rational Expressions Sequences Power Sums Pi Product Notation Induction.

Adding and subtracting with exponents. Multiply two numbers with exponents by adding the exponents together. Xm xn xm n. Divide two numbers with exponents by subtracting one exponent from the other.

Xm xn xm n. When an exponent is raised to a power multiply the exponents together. Xy z xy z.

Adding and Subtracting with Exponents When dealing with numbers only we look at each expression calculate and then add or subtract as needed. The addition problem 22 33 becomes 2 2 3. The same rules that apply to adding exponents apply to subtracting as well.

You can only subtract numbers that have unknowns with the same exponent. 4x12 025 x4 2x2 3x2 3x12 Solution. 4x12 3x12 025cdot x4 2x2 3x2 x12 025cdot x4 x2.

Remember to add or subtract numbers that have exponents you must first make sure that the base and exponent of the two terms you are trying to add or subtract are the same. If they are the same then all you have to do is add together their coefficients and keep the base and exponent the same. Lets look at another example.

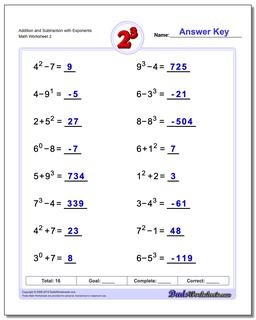
Adding and Subtracting Exponents. These exponent worksheets have addition and subtraction problems adding simple exponential terms to numbers as well as adding two exponential terms to each other. The problems in these worksheets help teach order of operations with exponents in a simple context.

Subtracting exponents with different base. 4 2 3 2 16 9 7. 11x 7y -2x 3x.

11x 2x 3x 7y. Evaluate 3x 2 7y 2. In this case the two exponents 3x 2 and 7y 2 are unlike terms and so it will remain as it is.

Here 3x and 7y both are unlike. Evaluate 15x 12y 11x 15x. Subtracting terms with fractional exponents follows the same rules as adding terms with fractional exponents.

The terms must have the same base a and the same fractional exponent nm. The rule is given as. Canm Danm C Danm.

To add or subtract with powers both the variables and the exponents of the variables must be the same. You perform the required operations on the coefficients leaving the variable and exponent as they are. When adding or subtracting with powers the terms that combine always have exactly the same variables with exactly the same powers.

When you find yourself with addition and subtraction of powers you should not be confused with adding or subtracting the exponents. Remember that the properties of the powers are not applicable with addition and subtraction only with multiplications and divisions. You can only add and subtract exponents when they are made of like terms That means that both the base and the power must be the same.

For example take the problem 2x2 3y x2 4y2. Scientific Notation - Adding And Subtracting Learn how to add and subtract in scientific notation. Add and subtract numbers in different bases by rewriting their exponents to be the same.

The basic rule in adding and subtracting variables with exponents is they must be like terms. Like terms consist of the same variable or set of variables raised to the same power. The numerical coefficients of these terms may vary and these are the elements that undergo the addition or subtraction process.

To add or subtract quantities in scientific notation numbers are manipulated so that they contain similar bases and exponents. This is done in order to ensure the corresponding integers in their coefficients are in the same place value. Using this review scavenger hunt to practice the skills of multiplying and dividing monomials or in simpler terms adding and subtracting exponents.

This scavenger hunt has varying problems allowing all levels of students to practice and to become successful with this concept. Order of Operations Factors Primes Fractions Long Arithmetic Decimals Exponents Radicals Ratios Proportions Percent Modulo Mean Median Mode Scientific Notation Arithmetics Algebra Equations Inequalities System of Equations System of Inequalities Basic Operations Algebraic Properties Partial Fractions Polynomials Rational Expressions Sequences Power Sums Pi Product Notation Induction. To subtract Polynomials first reverse the sign of each term we are subtracting in other words turn into - and - into then add as usual.

After subtracting 2xy from 2xy we ended up with 0 so there is no need to mention the xy term any more. When adding or subtracting numbers with exponents all we need to do is calculate each expression and solveIn order to add or subtract with variables you must have like bases and like exponents which means the expressions you are dealing with have the same bases and exponents. You can then add or subtract the coefficients in order to get an answer.

Once you have added or subtracted the coefficients you must remember that the base and exponent remain the same. In the special case where.