How to calculate elastic collision

Next measure the initial velocities of each objectUsing a speed radar or another formula calculate the initial. After they collide and assuming the collision is perfectly elastic the two objects will always depart at a right angle to each other.

The collision was elastic so kinetic energy was conserved.

How to calculate elastic collision. How to calculate an elastic collision. First determine the masses of each objectMeasure the masses of object 1 and 2 using an accurate scale or formula. Next measure the initial velocities of each objectUsing a speed radar or another formula calculate the initial.

Calculate the final. An elastic collision is a collision where both kinetic energy KE and momentum p are conserved. This means that KE 0 KE f and p o p f.

Recalling that KE 12 mv 2 we write 12 m 1 v 1i 2 12 m 2 v i 2 12 m 1 v 1f 2 12 m 2 v 2f 2 the final total KE of the two bodies is the same as the initial total KE of the two bodies. An elastic collision is one in which the total kinetic energy of the two colliding objects is the same before and after the collision. For an elastic collision kinetic energy is conserved.

05m 1 v i1 2 05m 2 v i2 2 05m 1 v f1 2 05m 2 v f2 2 The collision is fully specied given the two initial velocities and masses of the colliding objects. As in all collisions momentum is conserved in this example. But calculations comparing kinetic energy before and after the collision show kinetic energy is not conserved.

This is an inelastic. Inelastic Collision Formula When two objects collide with each other under inelastic condition the final velocity of the object can be obtained as. Elastic collision is used to find the final velocities v1 and v2 for the mass of moving objects m1 and m2.

V 1 m 1 - m 2 m 1 m 2 v 1 v 2 2m 1 m 1 m 2 v 1 Where m 1 m 2 - Mass of Moving Objects v 1 - Velocity of Moving Objects. Lets assume that we need to calculate the velocities V1 and V2 of the two masses after the elastic collision has taken place. The first step is to design the vectors of velocity for each of the bodies before and after the collision.

Choose the positive direction usually toward the right. The collision was elastic so kinetic energy was conserved. KE 12 mv2 so heres your equation for the two cars final and initial kinetic energies.

Now you have two equations and two unknowns vf1 and vf2 which means you can solve for the unknowns in terms of the masses and vi1. Equations for post-collision velocity for two objects in one dimension based on masses and initial velocities. V_1 fracu_1m_1-m_22m_2u_2m_1m_2 v_2 fracu_2m_2-m_12m_1u_1m_1m_2.

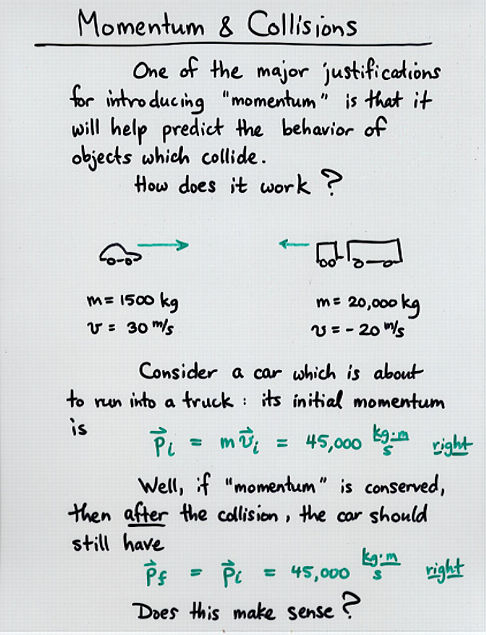
Determine the final velocity of one of the objects. For example we know that after the collision the first object will slow down to 4 ms. Calculate the momentum of the system before the collision.

In this case initial momentum is equal to 8 kg 10 ms 4 kg 0 ms 80 Ns. Collisions between hard spheres may be nearly elastic so it is useful to calculate the limiting case of an elastic collision. The assumption of conservation of momentum as well as the conservation of kinetic energy makes possible the calculation of the final velocities in two-body collisions.

Using the fact that you say the velocities can be interchanged we obtain a final answer of. M 1 m 2 v 2 v 1 v 2 v 1 This final step gives a ratio of 1 between the two masses. The simple calculator which is used to calculate the final velocities V1 and V2 for an elastic collision of two masses in one dimension.

Code to add this calci to your website Just copy and paste the below code to your webpage where you want to display this calculator. Calculating Momentum and Kinetic Energy Calculate the momentum and kinetic energy for each cart using the masses from part I and the velocities from part II. Remember to add the carts masses when the carts are stuck together.

Now add the results for the purple and green carts to determine the total momentum and kinetic energy before the collision. Elastic Collision Formula An encounter between two bodies in which the total kinetic energy of both the bodies after the encounter is equal to their total kinetic energy before the encounter is called Elastic collision. Elastic collisions occur only when there is no net conversion of kinetic energy into different forms.

Collision is to use this equation which says the initial velocity of one of the objects before the collision plus the final velocity of that same object after the collision should equal if its an elastic collision itll equal the initial velocity of the second object before the collision. After they collide and assuming the collision is perfectly elastic the two objects will always depart at a right angle to each other. We can prove this fact by applying the conservation of momentum physical law the conservation of energy true iff the collision is perfectly elastic and the law of cosines pure math.

After the hit the players tangle up and move with the same final velocity. Therefore the final momentum pf must equal the combined mass of the two players multiplied by their final velocity m1 m2 vf which gives you the following equation. M1 m2 vf m1vi1.