How to do factoring polynomials

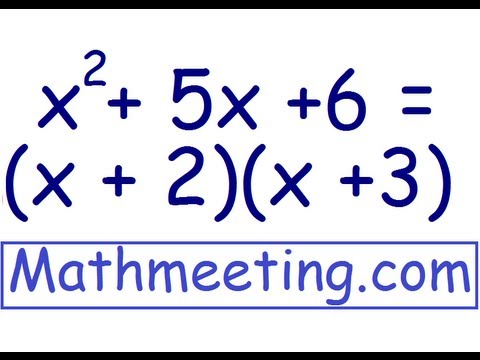
Set the equation to equal zero. The first method for factoring polynomials will be factoring out the greatest common factor.

Given a polynomial expression factor out the greatest common factor.

How to do factoring polynomials. Factoring polynomials can be easy if you understand a few simple steps. This video will explain how to factor a polynomial using the greatest common factor. The first method for factoring polynomials will be factoring out the greatest common factor.

When factoring in general this will also be the first thing that we should try as it will often simplify the problem. To use this method all that we do is look at all the terms and determine if there is a factor that is in common to all the terms. If there is we will factor it out of the polynomial.

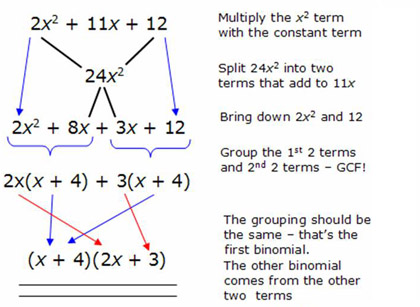
Check for the GCF first. The expressionwont have GCF when you break it down and look at it according to the steps in. Multiply the quadratic term and the constant term.

Be careful of the signs when you do this. In this example the. Write down all the factors of the.

How to Factor Polynomials 1 Take out the GCF if possible Learn how to factor out a GCF 2 Identify the number of termsMore information about terms 2 term factoring techniques 3 term factoring techniques 3 Check by multiplying. Follow the steps mentioned below to factorize a polynomial. Factor out if there is a factor common to all the terms of the polynomial.

Identify the appropriate method to factorize the polynomial. You can use regrouping or algebraic identities to find the factors of the polynomial. There are a few more terms that it is good to know when you are dividing polynomials.

Dividend - The polynomial you are factorisingdividing. In this case x2 7x 3 divisor - The number. Given a polynomial expression factor out the greatest common factor.

Identify the GCF of the coefficients. Identify the GCF of the variables. Combine to find the GCF of the expression.

Determine what the GCF needs to be multiplied by to obtain each term in the expression. Press the PRGM button scroll once to the right to IO scroll down and select ClrHome. Press PRGM again scroll once to the right to IO and select Input then hit 2ND ALPHA and type in ENTER A use the to make quotations.

After ENTER A put a comma followed by the variable A. The first step in solving a polynomial is to find its degree. The Degree of a Polynomial with one variable is.

The largest exponent of that variable. When we know the degree we can also give the polynomial a name. But what if we want to characterize or find some special way to express factor such a polynomial.

How can we do it. Polynomials optimization reference-request soft-question factoring. Follow asked 9 mins ago.

227k 9 9 gold badges 31 31 silver badges 76 76 bronze badges endgroup. Solving a Quadratic Polynomial 1. Determine whether you have a quadratic polynomial.

A quadratic polynomial is a polynomial of the second degree. Make sure the polynomial is written in order of degree. Set the equation to equal zero.

This is a necessary step for solving all polynomials. In this video you will learn how to factor a polynomial completely. The first step is to find the GCF or the greatest common factor of the polynomial.

So when a polynomial is written as a product of polynomials each of the polynomials is a FACTOR of the original polynomial. This implies that Factoring is the name given to the process of writing a polynomial as a product of polynomials. Understanding of another term would be needed to proceed with the factorization and that is GCF or HCF.

In general factoring will undo multiplication. Each term of 10x 5 has 5 as a factor and 10x 5 5 2x 1. To factor an expression by removing common factors proceed as in example 1.

3x is the greatest common factor of all three terms. Find one factor by making use of the Remainder Theorem Divide the polynomial by the factor we found thus giving us a simpler polynomial to work with Find one factor of the simpler polynomial and divide once again. Zeroes of polynomials when represented in the form of another linear polynomial are known as factors of polynomials.

After factorisation of a given polynomial if we divide the polynomial with any of its factors the remainder will be zero. Also in this process we factor the polynomial by finding its greatest common factor. Factoring is also the opposite of Expanding.

In the previous example we saw that 2y and 6 had a common factor of 2. But to do the job properly we need the highest common factor including any variables. The first method for factoring polynomials will be factoring out the greatest common factor.

When factoring in general this will also be the first thing that we should try as it will often simplify the problem. To use this method all that we do is look at all the terms and determine if there is a factor that is in common to all the terms.