Mean value theorem for integrals example

The top of the rectangle which intersects the curve f c is the average value of the function. Differentiation under the integral sign Theorem.

Compare f p q-p and p q f xdx.

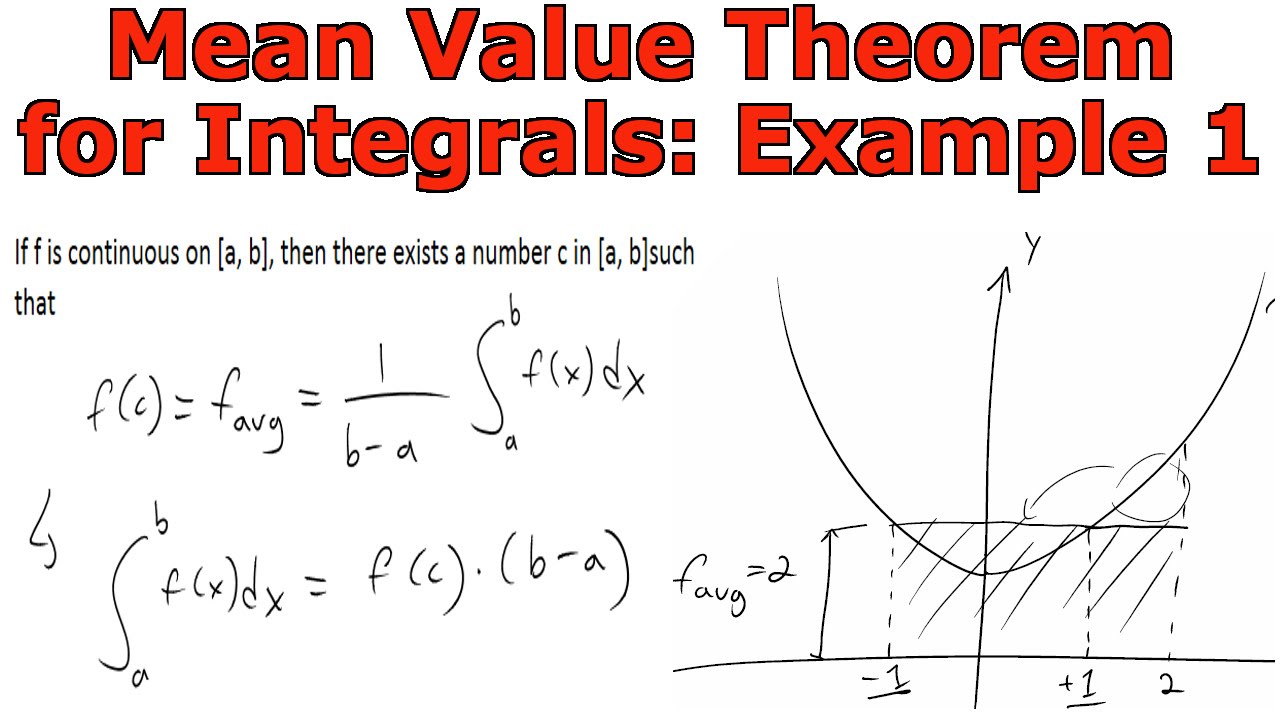
Mean value theorem for integrals example. Mean Value Theorem for Integrals. 28B MVT Integrals 2. Definition Average Value of a Function If fis integrable on ab then the average value of fon ab is EX 1 Find the average value of this function on 03 28B MVT Integrals 3 Mean Value Theorem for Integrals.

If fis continuous on ab there exists a valuecon the interval ab such that. Mean Value Theorems for Integrals Proof Example Mean value theorem defines that a continuous function has at least one point where the function equals its average value. Mean Value Theorems for Integrals Integration.

For example heres a figure that illustrates the definite integral. And its mean-value rectangle. Now heres how you calculate the average value of the shaded area.

Not surprisingly the average value of this integral is 30 a value between the functions minimum of 8 and its maximum of 64. Mean Value Theorem Example. Let fx 1x a -1 and b1.

We know fb fab-a 22 1. While for any cϵ -1 1 not equal to zero we have. Fc -1c 2 1.

Therefore the equation fc fb fa b a doesnt have any solution in c. But this does not change the Mean Value Theorem because fx is not continuous on -11. The Mean Value Theorem for integrals tells us that for a continuous function fx theres at least one point c inside the interval ab at which the value of the function will be equal to the average value of the function over that interval.

This means we can equate the average value of the funct. The MVT for integrals says that there exists a 𝑐 𝑎 𝑏 such that 𝑓 𝑐 is the average value of 𝑓 over 𝑎 𝑏 which we can quite easily disprove if 𝑓 is not continuous. Take the Heaviside function as an example.

𝐻 𝑥 0 for 𝑥 0. 𝐻 𝑥 1 for 𝑥 0. The Mean-Value Theorem For Integrals The average value of S1 1 2 3 is 1 2 33 2 which belongs to S1 while that of S2 1 2 9 is 1 2 93.

Assume the derivative of f at every interior point of the interval I exists and is zero. Let a b be an arbitrary open interval in I. By the mean value theorem there exists a point c in a b such that.

0 f c f b f a b a. Displaystyle 0f c frac f b-f a b-a. Assume that f x be continuous and increasing on pq.

Compare f p q-p and p q f xdx. Solution-The First Mean Value Theorem for Integrals implies the existence of r Є pq such that. F r q-p p q f x dx.

As f x is increasing then f p f rHence f p q - p f r q-p which implies. The Mean Value Theorem for Integrals states that for every definite integral a rectangle with the same area and width w b-a exists. The top of the rectangle which intersects the curve f c is the average value of the function.

Before we take a look at a couple of examples lets think about a geometric interpretation of the Mean Value Theorem. First define A af a A a f a and B bf b B b f b and then we know from the Mean Value theorem that there is a c c such that a c b a c b and that. Gt 2tt2 t3 g t 2 t t 2 t 3 on 21 2 1 Solution For problems 3 4 determine all the number s c which satisfy the conclusion of the Mean Value Theorem for the given function and interval.

Hz 4z3 8z2 7z 2 h z 4 z 3 8 z 2 7 z 2 on 25 2 5 Solution. Does The Mean Value Theorem For Integrals Always Hold In The Complex Plane. Provide Examples Of When The Theorem Does Hold And When It Doesnt If Possible.

This question hasnt been answered yet Ask an expert. Show transcribed image text. Previous question Next question.

Differentiation under the integral sign Theorem. Let fx t be a function such that both fx t and its partial derivative f x x t are continuous in t and x in some region of the x t-plane including ax t bx x 0 x x 1Also suppose that the functions ax and bx are both continuous and both have continuous derivatives for x 0 x x 1. This video explains and provide an example of a problem involving the mean value theorem for integrals.

A circumstance where the Mean Value Theorem for Integrals fails is when the function is integrable but discontinuous. The Mean Value Theorem for Integrals is f c 1 b a a b f x So if we want to prove it fails for a specific integral then I assume we would want to prove that f c takes on two different values. Lesson Overview The Mean Value Theorem for Integrals is a direct consequence of the Mean Value Theorem for Derivatives and the First Fundamental Theorem of Calculus.

In words this result is that a continuous function on a closed bounded interval has at least one point where it is equal to its average value on the interval.