Moving the points changes the limits of the inequalities. Translate the problem into a probability statement about X.
Standardize the x -value to a z -value using the z -formula.
Normal approximation to the binomial distribution. The selection of the correct normal distribution is determined by the number of trials n in the binomial setting and the constant probability of success p for each of these trials. The normal approximation for our binomial variable is a mean of np and a standard deviation of np 1 - p 05. For example suppose that we guessed on each of the 100 questions of a multiple-choice test where each question had one correct answer out of four choices.
In order to use the normal approximation we consider both np and n 1 - p. If both of these numbers are greater than or equal to 10 then we are justified in using the normal approximation. This is a general rule of thumb and typically the larger the values of np and n 1 - p the better is the approximation.
Normal Approximation to the Binomial Distribution. The continuous normal distribution can sometimes be used to approximate the discrete binomial distribution. This is very useful for probability calculations.
It could become quite confusing if the binomial formula has to be used over and over again. Hence normal approximation can make these calculation much easier to work out. Normal approximation is often used in statistical inference.
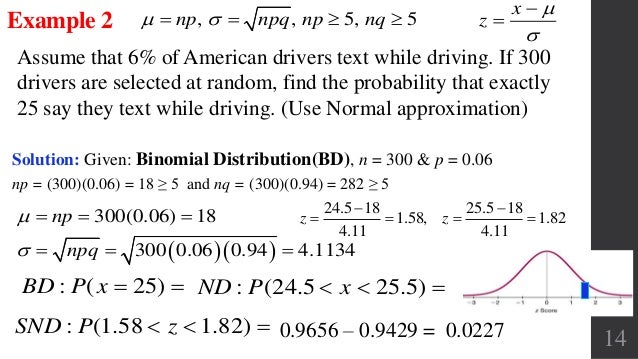
We can approximate X B20001 by the normal distribution Y N204242 and use the transformation Z Y 20 424 N01 so that P25 X 29 P245 Y 295 P24520 424 Z 29520 424 P106 Z 224 0487503554 01321 HELM 2008. The Normal Approximation to the Binomial Distribution. The general rule of thumb to use normal approximation to binomial distribution is that the sample size n is sufficiently large if np 5 and n1 p 5.
For sufficiently large n X Nμ σ2. That is Z X μ σ X np np 1 p N0 1. Continuity Correction for normal approximation.
So go ahead with the normal approximation. Translate the problem into a probability statement about X. In this example you need to find p X 60.
Standardize the x -value to a z -value using the z -formula. For the mean of the normal distribution use. The mean of the binomial and for the standard deviation.
Therefore the Poisson distribution with parameter λ np can be used as an approximation to Bn p of the binomial distribution if n is sufficiently large and p is sufficiently small. According to two rules of thumb this approximation is good if n 20 and p 005 or if n 100 and np 10. X B30 04 b Use the normal distribution to calculate an approximation for PX 8 c Use the binomial distribution to find PX 8 d Calculate the percentage error in the approximation found in part b.
Normal approximation to the binomial distribution. The approximation concept becomes crucial when the computational difficulties began to arise when a binomial formula is used. Learn how to use the Normal approximation to the binomial distribution to find a probability using the TI 84 calculator.
Using the normal approximation to the binomial distribution simplified the process. To compute the normal approximation to the binomial distribution take a simple random sample from a population. You must meet the conditions for a binomial distribution.
There are a certain number n of independent trials. Binomial Distribution The parameters for the normal and binomial distributions can be set with the sliders. Moving the points changes the limits of the inequalities.
By using a continuity correction the approximation is more accurate. The general rule of thumb to use normal approximation to binomial distribution is that the sample size n is sufficiently large if n p 5 and n 1 p 5. For sufficiently large n X N μ σ 2.
That is Z X μ σ X n p n p 1 p N 0 1. Adjust the binomial parameters n and p using the sliders. Click Overlay normal to show the normal approximation.
Click Show points to reveal associated probabilities using both the normal and the binomial. Drag the points on the X-axis to change the areas. The normal approximation tothe binomial distribution Remarkably when n np and nq are large then the binomial distribution is well approximated by the normal distribution.
83 on p762 of Boas fx Cnxpxqnx 1 2πnpq exnp22npq. In these notes we will prove this result and establish the size of.