Partial sum of arithmetic sequence

The formula for the first n terms of an arithmetic sequence starting with i 1 is. The partial sum is certainly that.

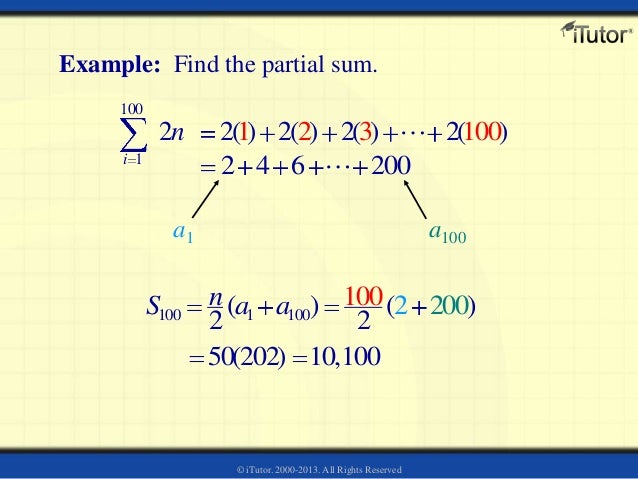
The cumulative sum of the first n terms of an arithmetic sequence is given by the number of terms involved times the average of the first and.

Partial sum of arithmetic sequence. The k th partial sum of an arithmetic series is You simply plug the lower and upper limits into the formula for an to find a1 and ak. Arithmetic sequences are very helpful to identify because the formula for the n th term of an arithmetic sequence is always the same. An a1 n 1 d.

You can also sum numbers on the sequence up to a certain index n which is called partial sum. The formula for the partial sum would be. But you can also sum these partial sums as well.

This is what the calculator below does. You enter the first term of the sequence the common difference and the last index to compute and the calculator displays the table with the following columns. I-th member of the sequence.

This is the Partial Sum of the first 4 terms of that sequence. A Sequence is a set of things usually numbers that are in order. A Partial Sum is the sum of part of the sequence.

The sum of infinite terms is an Infinite Series. And Partial Sums are sometimes called Finite Series. A sequence of partial sums of the series S is a sequence where each term is the nth partial sum of S.

In simple terms in allows you to work with infinite sums eg. 1 2 which would be impossible to evaluate without some kind of cutoff point. However where a sequence of partial sums is really important is because of what it tells us about its parent sequence.

The partial sum is certainly that. However we are given the formula for the sum. So instead of writing a1 a2 a3 a4 a5 a6 we could write Sn.

Since were looking for the partial sum of the first six we can just plug in 6 into Sn and find the actual sum of the first six terms. Discrete Math 914 PART C. FORMULA FOR THE nth PARTIAL SUM OF AN ARITHMETIC SEQUENCE The nthpartial sum of an arithmetic sequence with initial term a 1 and common difference d is given by.

S n n a 1 a n 2 Think. The cumulative sum of the first n terms of an arithmetic sequence is given by the number of terms involved times the average of the first and. Using the Arithmetic Sequence rule.

X n a dn1 3 5n1 3 5n 5 5n 2. So the 9th term is. X 9 59 2 43.

S n 2 a 1 a 1 n 1 d And finally it will be. S n 2 2 a 1 n 1 d Now this formula will provide help to find the sum of an arithmetic sequence. The distance formula has different concepts than arithmetic sequenc formula.

For learning distance formula equation use Distance Formula Calculator. The finite partial sums of the diverging harmonic series are called harmonic numbers. The difference between Hn and ln n converges to the EulerMascheroni constant.

The difference between any two harmonic numbers is never an integer. Free Arithmetic Sequences calculator - Find indices sums and common difference step-by-step This website uses cookies to ensure you get the best experience. By using this website you agree to our Cookie Policy.

For instance a sequence has a total of 10 terms but were given the sum of the first two terms only. We have a partial sum sum of only the first two terms of the sequence. At times were asked to find the sum of the sequence using the partial sum.

So how do we solve such problems. The formula to compute the next number in the sequence is You can also sum numbers on the sequence up to certain index n which is called partial sum the formula for the partial sum would be But you can also sum these partial sums as well. This is what the calculator below does.

For reasons that will be explained in calculus you can only take the partial sum of an arithmetic sequence. The partial sum is the sum of a limited that is to say a finite number of terms like the first ten terms or the fifth through the hundredth terms. The formula for the first n terms of an arithmetic sequence starting with i 1 is.

A partial sum of an infinite series is the sum of a finite number of consecutive terms beginning with the first term. When working with infinite series it is often helpful to examine the behavior of the partial sums. Suppose an infinite sequence is defined by.

The terms of the sequence are. Cesàro summation is an averaging method in that it relies on the arithmetic mean of the sequence of partial sums. Other methods involve analytic continuations of related series.

In physics there are a wide variety of summability methods. These are discussed in greater detail in the article on regularization.