Standard deviation of binomial

Standard deviation of binomial distribution. 95 CI of SD can be.

What is the probability of getting exactly 3 times head.

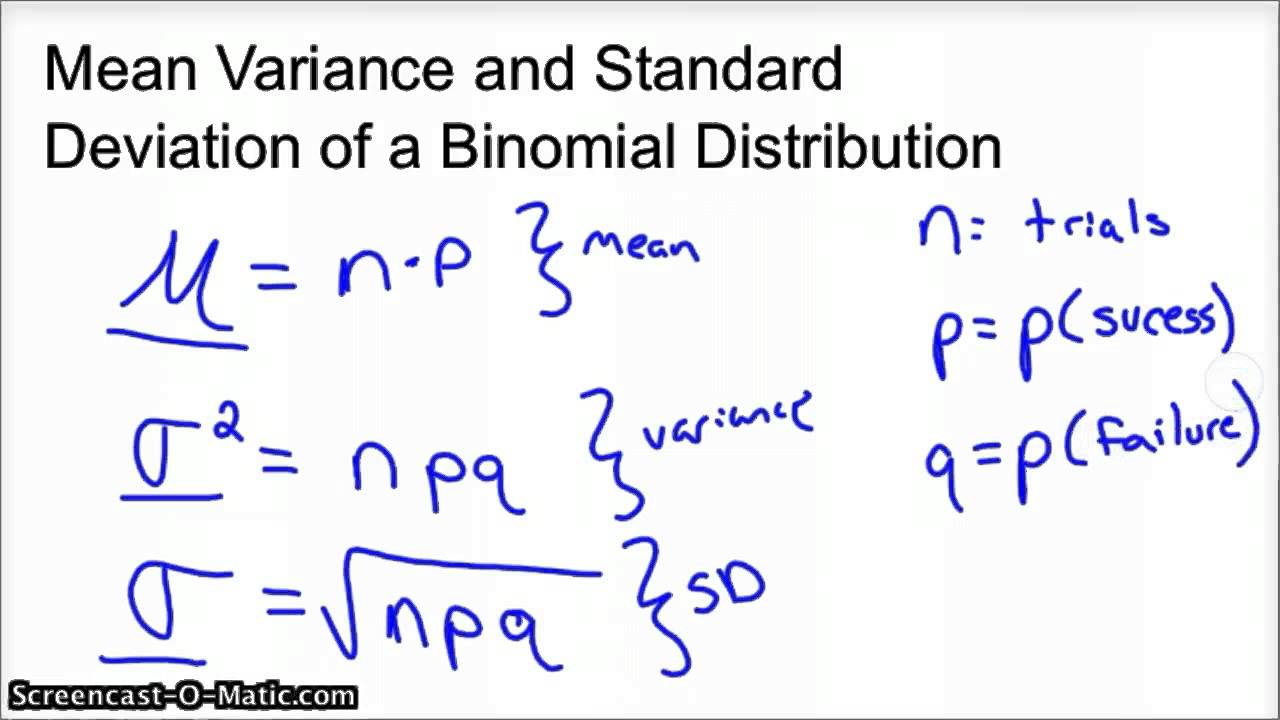
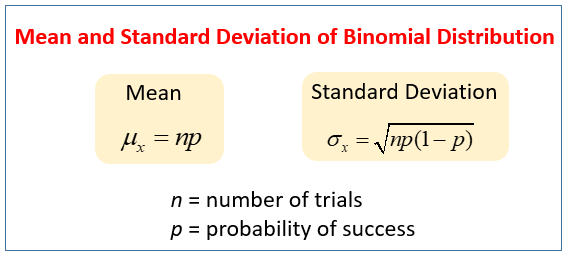
Standard deviation of binomial. For a Binomial distribution μ the expected number of successes σ 2 the variance and σ the standard deviation for the number of success are given by the formulas. μ n p σ 2 n p q σ n p q Where p is the probability of success and q 1 - p. Mean and Standard Deviation for the Binomial Distribution.

The binomial probability is a discrete probability distribution with appears frequently in applications that can take integer values on a range of 0 n for a sample size of n. The population mean is computed as. Mu n cdot p Also the population variance is computed as.

Standard deviation of binomial distribution. Standard deviation is also a standard measure to find out how spread out are the no. From the mean value.

Standard Deviation Variance 12 npq 12. A coin is tossed five times. What is the probability of getting exactly 3 times head.

Also find mean variance and standard deviation. If you repeat the experience a large number of times testing 500 chips approximately 68 of the sample means will be between -31310 and 31310. Comment on John Smiths post Mean.

On average 10 out of 500 chips will be defe. 2 votes Button opens signup modal. The standard deviation of X is.

For example suppose you flip a fair coin 100 times and let X be the number of heads. Then X has a binomial distribution with n 100 and p 050. Heads which makes sense because if you flip a coin 100 times you would expect to get 50 heads.

The variance of X is. Deviation of Binomial Distribution Formula σ npq n number of trials p probability of success q probability of failure q 1 - p. The standard deviation of X is represented by and represents the square root of the variance.

If X has a binomial distribution the formula for the standard deviation is where n is the number of trials and p is the probability of success on each trial. Standard Deviation is an interval estimate. But how precise is your estimation of sample SD that plots scatter or variability of data in comparison with the population.

95 CI of SD can be. In probability theory and statistics the binomial distribution with parameters n and p is the discrete probability distribution of the number of successes in a sequence of n independent experiments each asking a yesno question and each with its own Boolean-valued outcome. Success with probability p or failure with probability q 1 pA single successfailure experiment is also.

The calculator will find the binomial and cumulative probabilities as well as the mean variance and standard deviation of the binomial distribution. Show Instructions In general you can skip the multiplication sign so 5x is equivalent to 5x. Standard deviation of binomial distribution calculator uses Standard DeviationsqrtNumber of trialsProbability of Success1-Probability of Success to calculate the Standard Deviation The Standard deviation of binomial distribution formula is definedby the formula SD square root of n P 1 - P.

Where n is the number of trails and P is the probability of successful outcome. What is the formula of finding the standard deviation of a binomial distribution. Asked Aug 12 2019 in Data Science by Tedsf Diamond 52260 points 136 views standard-deviation.

If mean and standard deviation of a binomial distribution is 10 and 3 respectively. Q will be ___ a 03 b 033 c 30 d 09 Quantitaive Aptitude Business Statistics. Probability Distributions 37 4.

The standard deviation of the binomial distribution is equal to 3795. Become a member and unlock all Study Answers. Try it risk-free for 30 days Try it risk-free Ask a question.

In many cases it is not possible to sample every member within a population requiring that the above equation be modified so that the standard deviation can be measured through a random sample of the population being studied. A common estimator for σ is the sample standard deviation typically denoted by s. The standard deviation of the estimator is the square root of the variance so it is p 1 p N.

So if you throw a coin 100 times and you observe 49 heads then 49 100 is an estimator of for the probability of tossing head with that coin and the standard deviation of this estimate is 049 1 049 100. The standard deviation of the binomial distribution is interpreted as the standard deviation of the number of successes for the distribution. To find the standard deviation use the formula σ n p1 p σ n p 1 p where n is the umber of trials and p is the probability of success on a single trial.

The formula for a binomial distribution standard deviation solved step by step.