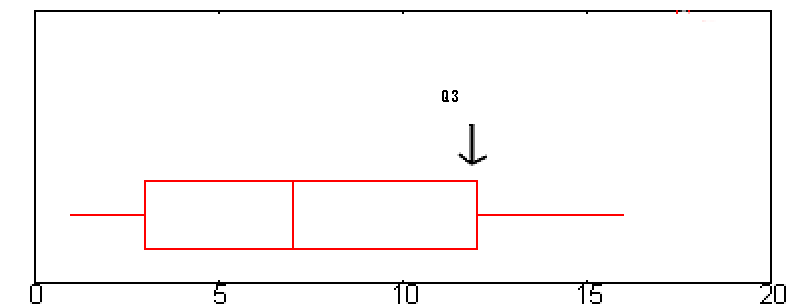
Image will be. The values that divide each part are called the first second and third quartiles.

One popular way to measure spread is the interquartile range which is calculated as the difference between the first quartile and the third quartile in a dataset.
What is iqr in statistics. In descriptive statistics the interquartile range IQR also called the midspread middle 50 or Hspread is a measure of statistical dispersion being equal to the difference between 75th and 25th percentiles or between upper and lower quartiles IQR Q 3 Q 1In other words the IQR is the first quartile subtracted from the third quartile. These quartiles can be clearly seen on a. The interquartile range IQR is the length of the middle 50 of that interval of space.
The interquartile range is the middle 50 of a data set. Box and whiskers image by Jhguch at enwikipedia. The interquartile range IQR is the range of values that resides in the middle of the scores.
When a distribution is skewed and the median is used instead of the mean to show a central tendency the appropriate measure of variability is the Interquartile range. The interquartile range IQR is the difference between the first quartile and third quartile. The formula for this is.
IQR Q 3 - Q 1 There are many measurements of the variability of a set of data. In descriptive statistics the interquartile range tells you the spread of the middle half of your distribution. Quartiles segment any distribution thats ordered from low to high into four equal parts.
The interquartile range IQR contains the second and third quartiles or the middle half of your data set. The interquartile range IQR is a measure of variability based on dividing a data set into quartiles. Quartiles divide a rank-ordered data set into four equal parts.
The values that divide each part are called the first second and third quartiles. And they are denoted by Q1 Q2 and Q3 respectively. IQR or InterQuartile Range is probably one of the most widely taught statistics in introductory courses however it is in our opinion one of the least utilized in analysis.
The interquartile range IQR is a measure of variability based on dividing a data set into quartiles. The values that divide each part are called the first second and third quartiles. And they are denoted by Q1 Q2 and Q3 respectively.
The IQR is the interquartile range of a data set. It is used in statistical analysis to help draw conclusions about a set of numbers. The IQR is often preferred over the range because it excludes most outliers.
Read on to learn how to. In statistical dispersion Interquartile range IQR is the measurement of difference between the third and the first quartiles. Mathematically it is obtained when the 1st quartile is subtracted from the 3rd quartile.
IQR is otherwise called as midspread or middle fifty. It is expressed as IQR Q 3 - Q 1. In statistics were often interested in knowing how spread out the values are in a distribution.
One popular way to measure spread is the interquartile range which is calculated as the difference between the first quartile and the third quartile in a dataset. Quartiles are simply values that split up a dataset into four equal parts. The IQR measures the variability of a distribution by giving us the interval covered by the middle 50 of the data.
The five-number summary of a distribution consists of the minimum quartile 1 median quartile 3 and maximum. The IQR is the measure of spread we should use when using the median to measure center. The IQR equals Q3 Q1 that is the 75th percentile minus the 25th percentile and reflects the distance taken up by the innermost 50 of the data.
If the IQR is small you know the data are mostly close to the median. If the IQR is large you know the data are more spread out from the median. Interquartile range is the difference between the upper quartile and the lower quartile.
The interquartile range formula is given below. Interquartile range - Upper quartile- Lower quartile Q₃ - Q₁ In the above interquartile range formula Q₁ represents the lower quartile whereas Q₃ represents the upper quartile. Image will be.
The interquartile range often denoted IQR is a way to measure the spread of the middle 50 of a dataset. It is calculated as the difference between the first quartile Q1 and the third quartile Q3 of a dataset. Quartiles are simply values that split up a dataset into four equal parts.
For example suppose we have the following dataset. The interquartile range is the third quartile Q3 minus the first quartile Q1. This gives us the range of the middle half of a data set.
To find the interquartile range of your 8 data points you first find the values at Q1 and Q3. Multiply the number of values in the data set 8 by 025 for the 25th percentile Q1 and by 075 for the 75th percentile Q3.