Additionally if a radius of a circle is perpendicular to a chord then the radius bisects the chord. A chord is a straight line joining 2 points on the circumference of a circle.

We discuss a few of them here as they often prove helpful in solving various questions.
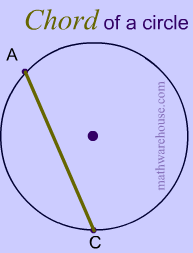
What is the chord of a circle. Chord of a Circle Definition The chord of a circle can be defined as the line segment joining any two points on the circumference of the circle. It should be noted that the diameter is the longest chord of a circle which passes through the center of the circle. The figure below depicts a circle and its chord.
By definition a chord is a straight line joining 2 points on the circumference of a circle. The diameter of a circle is considered to be the longest chord because it joins to points on the circumference of a circle. In the circle below AB CD and EF are the chords of the circle.
Chord CD is the diameter of the circle. The chord is the line going across the circle from point A you to point B the fishing pier. The circle outlining the lakes perimeter is called the circumference.
A chord of a circle is a line. Additionally if a radius of a circle is perpendicular to a chord then the radius bisects the chord. The converse is also true.
If one chord is a perpendicular bisector of another chord then the first chord is a radius. The same also holds if instead of a radius its a diameter as evidenced by the diagram to the right. A chord of a circle is any line segment joining two points on the circumference of the circle.
What Are Equal Chords in a Circle. Chords that are equidistant from the center of a circle are considered equal chords. Now we will discuss some other fundamental properties related to circles.
A chord of a circle is a straight line segment whose endpoints both lie on a circular arcThe infinite line extension of a chord is a secant line or just secantMore generally a chord is a line segment joining two points on any curve for instance an ellipseA chord that passes through a circles center point is the circles diameterThe word chord is from the Latin chorda meaning bowstring. The chord of a circle is defined as the line segment that joins two points on the circles circumference. We can say that the diameter is the longest chord of a circle.
The diameter is a line segment that joins two points on the circumference of a circle which passes through the centre of the circle. Scroll down the page for examples explanations and solutions. A chord is a straight line joining 2 points on the circumference of a circle.
A radius or diameter that is perpendicular to a chord divides the chord into two equal parts and vice versa. In the above circle if the radius OB is perpendicular to the chord PQ then PA AQ. The diameter would be the longest chord in the circle.
The radius of the circle is a line segment from the center of the circle to a point on the circle. The plural of radius is radii. In the above diagram O is the center of the circle and and are radii of the circle.
The radii of a circle are all the same length. The theorem says that. Any line drawn from the center that bisects a chord is perpendicular to the chord.
Your statement is missing the word line and also it will be perpendicular to the chord instead of circles centre. Chord length 2r 2 -d 2 where r is the radius of the circle and d is the perpendicular distance of the center of the circle to the chord. There are various important results based on the chord of a circle.
We discuss a few of them here as they often prove helpful in solving various questions. Let R be the radius of the circle θ the central angle in radians α is the central angle in degrees c the chord length s the arc length h the sagitta of the segment and d the height or apothem of the triangular portion. The radius is The radius in terms of h and c can be derived above by using the Intersecting Chords Theorem where 2R the diameter and c are perpendicularly.
A chord is any line segment whose endpoints lie on a circle. The diameter is a special kind of chord that passes through the center of a circle. It is also the longest possible chord for a given circle.
In the diagram above line segment AB and CD are both chords. A chord of length 16 cm is drawn in a circle of radius 10 cm. Find the distance of the chord from the centre of the circle.
AB is a chord of length 16 cm. C is the midpoint of AB. OB is the radius of length 10 cm.
AB 16 cm. Chord of a Circle Definition The chord of a circle can be defined as the line segment joining any two points on the circumference of the circle. It should be noted that the diameter is the longest chord of a circle which passes through the center of the circle.
Theorem on Chord Properties. A chord is the line segment that joins two different points of the circle which can also pass through the centre of the circle. If a chord passes through the centre of the circle then it becomes diameter.
Suppose here we consider d as the diameter then the radius is given by d r2. The formula for the radius of a circle based on the length of a chord and the height is. R L2 8h h 2 r L 2 8 h h 2.